Notes on mathematical inhibitionby Jorge Canestri and Silva OlivaA) PREMISE The whole range of spontaneous reactions of people - whether learned or not - can have when faced with the need to understand or perform abstract mental operations requiring the use of mathematical formalisms, is perfectly comprehensible and needs no further explanation. In today's culture, there is perhaps a greater awareness of the enormous deficiency of this statement and of its consequences. As authors of these notes, and in our alternating and successive roles of mathematicians, teachers and psychoanalysts, we have chosen to deal with this topic by taking examples from different fields - some from our teaching experiences and others from our clinical practice. We are speaking of difficulties that are common to many people and, for two reasons, are especially apparent during school years. The first reason is that this is the specific period of development of the cognitive structures, and the second is due to the simple fact that these structures come into contact with the subject-matter in question. The mathematics that is learned in primary school requires the comprehension of only a small number of symbols, the necessarily mechanical memorisation of the algorithms of the rational operations, and the recognition of certain elementary geometric forms; its difficulty index, therefore, is lower than that of reading a text (DSM III R). The difficulties that we are referring to relate to the mathematics taught in middle school and high school. We are obviously not including those people who are particularly familiar with the subject of mathematics whether for work or pleasure, and who have progressed beyond the average level taught in schools. From our everyday and professional experience, we have learned that these difficulties are also common among the adult population who have already passed their school years. The difference between these people and the school-age population is that the latter, unlike the former, are unable to avoid the exigencies of learning and using mathematical notions. Our experience also suggests that the manner in which a person confronts the world of mathematical abstraction is precisely that of avoiding the confrontation. We shall later consider what names, characteristics and motivations could correspond to this1 general act of withdrawal that we hypothesise. From a brief review of the psychoanalytic literature, we have noticed that the topic as we have presented it does not appear to have been taken into consideration. On the other hand, there are several works dealing with mathematical "talent" and with the development, genesis and metapsychology of abstract thinking (V.H. Rosen, 1953), with the specific narcissism of the mathematician (R. Fine and B. Fine, 1977), and with the relationships between mathematics and the unconscious and the pre-conscious (S. Ferenczi, J. Lacan, I. Matte Blanco), etc. For this reason we are limiting our discussion to a much narrower field; we are enquiring into the obstacles faced by the subject when confronting mathematics at school, and that can appear not only during the scholastic period but also later on in life. Although the subjects in question are not mathematicians or individuals who are particularly talented in mathematics, nor are they mentally retarded or suffering from any evident handicaps, the population that we are dealing is numerous and the difficulty is widespread. The American mathematician John Allen Paulos (1988) invented the neologism innumeracy meaning mathematical illiteracy, and wrote the book "Innumeracy. Mathematical illiteracy and its consequences" for the purpose of analysing the devastating effects that it has on thinking. Douglas Hofstadter regards it as a serious illness, and Sheila Tobias, author of another book on the same topic, speaks of it as "mathematical anxiety". Among the causes of this mathematical illiteracy, leading to rejection and anxiety, Paulos lists a poor and incorrect scholastic education, psychological blocks, and romanticised misunderstandings about the nature of mathematics. For three reason, we have focused our notes on what Paulos calls "psychological blocks" . The first reason is obvious inasmuch as we are attempting a psychoanalytical reflection on the problem. Secondly, although we recognise that there are defects in the education received in our own (and in other) schools, our experience teaches us that even though the basic quality of teaching may be improved and the availability and efforts of teachers increased, the problem may recede but does not disappear. Thirdly, Paulos (as well as other mathematicians) paradoxically thinks that these blocks are "natural", apparently without wondering about the reason that justifies them. He writes that: "· some of the blocks to dealing comfortably with numbers and probabilities are due to quite natural psychological responses to uncertainty, to coincidence, or to how a problem is framed. Others can be attributed to anxiety..." (page 4). The question that arises, therefore, is to understand why mathematics with its specific characteristics as a form of thinking, is able to generate in the subject anxiety, psychological blocks, and efforts to avoid having to do it. In the Introduction to his book Capire una dimostrazione (To understand a demonstration), Gabriele Lolli (1988) says: "Defining mathematics is difficult; all the philosophers have tried in vain, while to the mathematician a definition appears to be superfluous; he does not need it for his work." (Our translation.) There would therefore be no point in the authors trying to define it; but because the reader is not a working mathematician, we must explain at least some of the characteristics that are fundamental in provoking psychological responses. Abstraction, in its widest sense, is certainly a universally recognised characteristic of mathematical thinking, although it is true that many other disciplines also reach high levels of abstraction. But as Lolli (op. cit. p.192) says, the type of abstraction associated with mathematics seems to speak of a reality that is distinct from physical reality, although equally objective; a reality that has no direct correspondence with the world of the senses. He reminds us of Leibniz' project: " ... the mind will be liberated from having to think directly about things in themselves, but nevertheless everything will come out correctly." (Our translation.) Moreover, it is a type of language that is completely devoid of semantic values, and that relies on the quality of the informative syntax. It is this formalism that distinguishes the modern orientation of this discipline and its alliance with logic. Perhaps this brief description has already given an idea of some of the motives that could be at the origin of the difficulties experienced by certain subjects. In the first place, we can perceive that the separation from the physical reality of the senses that has always been a substantial condition of mathematical thinking (e.g.. Pascal: "always mentally substitute the thing that is defined with the definition", a concept also present in Aristotle) has been accentuated in the mathematics of this century. The history of mathematics (see Eléments d'histoire des mathématiques by Nicolas Bourbaki, 1969) shows this progressive and consistent alliance between axiomatic method, linguistic formalism and logic in contemporary mathematics. As we know, the thinking of the mathematician (as opposed to the everyday user) as he works does not strictly follow these general methodological rules, but they acquire importance during the demonstration and communication phase. The rules, however, do reach the user, although in a form that each time is adapted to the level of learning. The more or less romanticised accounts of the creative activity of many mathematicians (form Poincaré, to Kac, Hardy) provide pleasant and useful reading to demonstrate this statement. Secondly, the "divorce" that has taken place between mathematics and psychology is fairly evident although little recognised. There are of course exceptions such as those mathematicians or logicians who have enquired into the relationship between methods of thinking during mathematical-logical demonstration or validation, and the thought processes of an ordinary person when he has to solve a problem. Perhaps the most valid and interesting aspect of Philip Johnson-Lairdās research in the field of cognitive psychology (cfr. Mental Models, 1983) is precisely the demonstration of the difference in procedure between ordinary problem-solving and logical-mathematical problem-solving. Another exception is the above-mentioned Lolli, who deals with this problem in his book on demonstration, and hypothesises that an error at the level of psychological concept may have negative consequences at the theoretic or didactic level. We agree with this hypothesis and, inderectly, we hope that these notes of ours, as well as throwing some light on the reasons for "mathematical anxiety", might also contribute to the battle against mathematical illiteracy.
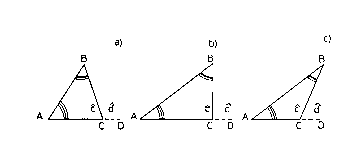
B) CHARACTERISTICS OF MATHEMATICS "How is it that mathematics, Albert Einstein, 1920. We can say that we are talking about mathematics when we abandon simple counting and begin searching for a shorter method for obtaining a result through a demonstration of "correctness": "demonstration generally marks the passage to real mathematics from a propedeutic phase of acquisition of ability and notions that are called mathematical, but that are only an extension of the physical mastery of the external environment, and that serve to better control the same" (Quotation from Lolli, p.9.) (Our translation) This is why the difficulties that we are referring to relate to mathematics as it is taught in middle and high schools. Beyond the level of primary school, specific difficulties arise owing to the fact that mathematics becomes an autonomous language that maintains its links with normal language, but is in the form of an artificial language, the learning of which is not supported or motivated by immediate everyday communication. It appears to be a kind of "dead language" whose terms have a meaning independent from the usual meaning of the words that we pronounce, and whose justification is obscure at the immediate time. This is why, at first encounter, mathematical language seems to be an authoritatively imposed necessity. Because of this "dogmatic" character, mathematical language is often thought of with dislike and is accused of being a cryptic language decipherable only by "experts". This phenomenon derives precisely from the "economic" character of mathematics, which is also manifest in the language used. One demonstration appears to be preferable to another because of the "essential rapidity" with which it achieves its aim. Avoiding the superfluous thus becomes an aesthetic characteristic (elegance) and therefore much sought after. The words used in the statements, as well as being essential because they contain a specific meaning, are also kept to the bare minimum; the final simplicity of the statements about theorems can be so rigorous and "Spartan" that it renders them completely incomprehensible to the average student. An interesting example of this is to present a group of students with the statement of any geometric or algebraic theorem and ask them to write only the hypothesis and the thesis, completely leaving out the demonstration. This exercise that consists simply in understanding the meaning of a sentence, often turns out to be extremely difficult and may require a surprisingly long period of "training". Another interesting example is to propose the following theorem which forms part of the normal programme of the 1st and 2nd years of high school. 1)In any given triangle, each external angle is greater that the non-adjacent internal angles. Whether or not the student knows how to demonstrate this theorem, a second statement can then be added: (2) In any given triangle, each external angle is greater than the internal angles. The question then asked is: What is the difference between the two statements? Are they both true, or only one of them? Even after learning how to demonstrate the first (and correct) statement, many students do not see any substantial difference between the two sentences. Or if they do, they cannot think of any reason for inserting "non-adjacent" in the first statement and not in the second, let alone provide simple examples that will enable them to decide which of the two is "right".
We can see from Figures (b) and c) that in the right-angled triangle and in the obtuse-angled triangle, the external angle is respectively equal to or smaller than adjacent angle C. However, there remains the suspicion that so much precision is a useless and pedantic waste of time, and that, on the whole the language of mathematics is somewhat persecutory. Let us look more carefully at the most obvious characteristic of mathematics - its abstraction. We take as an example the first introductory notions to a study of Euclidian geometry: the point, the line, the surface. These are the so-called "basic geometric entities" that provide the basis on which the whole geometric edifice is constructed. Generally, a point is drawn on the blackboard, and the teacher explains that the material point that everyone can see is not the geometric point; this latter, as well as being completely imaginary and invisible, must be thought of as being "dimensionless". The geometric point, therefore, can never be "experienced" but only imagined by divesting the material point of all those elements that make up its sensorial reality. The same process is repeated when passing on to the concept of line or surface, whose concrete shapes are drawn on the blackboard:
As in Magritte's La Trahison des Images, 1929, they are not the geometric "line" and "surface". These are pure conceptualizations obtained by depriving the concrete forms to which they refer of all the elements that we can see with our own eyes and that, for this very reason, we must ignore.
Another difficulty regards the dimensions of these two entities - length and breadth respectively. They must be thought of as being infinite - a concept that is quite inconceivable to the human mind. Moreover, the strength of geometry lies in the fact that the conclusions drawn are valid for any imaginable figure different from the figure that is used for carrying out the demonstration that, because it is concrete and individualised is not probative and is irrelevant. But we are more or less obliged to draw a concrete figure - for example, an obtuse-angled triangle that because it is such, cannot be acute-angled or right-angled - and to carry out a demonstration deliberately ignoring the fact that we are dealing with a triangle with precise physical characteristics. It is therefore necessary to operste a deliberate abstraction from the concrete object, and to deny its corporeal existence. To go one step further, the process of learning mathematics requires not only a capacity for abstraction or conceptualisation, but also a more sophisticated capacity for formalisation that is not called for in the same way in the ordinary thought capacities of everyday life or in the other sciences. The capacity for abstraction can be defined as the capacity for uniting in the same conceptual category different elements having the same common attributes, thus establishing the possibility of calling them by the same name; while the capacity for formalisation is the capacity to think of structures or abstract elements whose attributes are unknown or in any case of no account, and are identified solely on the basis of their "behaviour" namely their "form", i.e. a group of connections or links, compared with other elements or structures in the same condition. These "behaviour" or "forms" are described by mathematical language that possesses certain characteristics seemingly in contrast with the commonly-used meaning, as we have tried to show above, and that in any case has to be learned. Thus, after learning that 23 = 2 . 2 . 2 it should be possible - although it is not at all obvious - to easily work out x3 = x . x . x or (a+b)3 = (a+b) . (a + b) . (a + b) and lastly f3 (x) = f (x) . f (x) . f (x) which has a completely different meaning from f (x3). It seems to us, therefore, that the thought operations described above are in part completely peculiar to the discipline of mathematics, and often decidedly in contrast with the normally accepted needs of a common empirical mode of thought. The absolute character of this "anti-experientiality" of mathematics can be emphasised by comparing it with an example taken from the science of physics. We know that because of air resistance it is not possible to empirically prove the law according to which: "At any point on the Earth, the gravity acceleration is constant for all bodies, and is independent of their mass". Nevertheless, there is a piece of apparatus (Newton's tube) that in certain conditions enables us to experience the evidence of this law at the emotional level as well. Nothing of this kind is conceivable in mathematics, whose statements are judgements of truth based on logical inferences completely detached from the world of objects. Basically, the aim - as well as the challenge - of mathematics lies in devising and developing sophisticated formal languages to describe sensorially perceivable events in order to eliminate, in their communication, their restrictive dependence on current language and on other ordinary method of experiential transmission such as images, graphics, etc. We may recall the revolution that followed the invention of the method of Cartesian coordinates, enabling enormous steps to be made in algebraic geometry. To quote one of the simplest examples, it became possible to find out the mutual positions of two coplanar straight lines simply by calculations without using a graph. Mathematics cannot be considered as one of the natural sciences, nor can it be included among the humanistic disciplines invented by Man to describe his thoughts and emotions. However, inasmuch as it is a language - even though extremely sophisticated and formal - it is an invention and does possesses some characteristics in common with the humanistic disciplines. But because it does not convey the subject's contents, and because it is governed by strict regulations that make it suitable for describing the contents of the scientific disciplines, it shares the definition of these latter disciplines. It is therefore located in an intermediate area between the invention and the discovery of the invention itself. The only object that mathematics investigates is mathematics, by seeking to construct an apparatus for dealing with the consequences of the initial invention - the mathematical entities. Basically, it is a kind of "self-reflexive" discipline, and its "ambiguous" nature is responsible for some of the difficulties that we come across in trying to understand, for example, the so-called "proof by contradiction" (reductio ad absurdum). This consists in considering a statement (A) expressed in the thesis as "true" and therefore proved, because its opposite (non-A) contradicts a statement contained in the hypothesis. The hypothesis, however, has no claim to objective accuracy that can be experienced outside the subject; it is a pure conventionalism that becomes "true" because we all agree that it is so. The combination of all the hypotheses and all the truths forms the "reality" outside the subject, against which the subject must measure himself and on the basis of which he makes his judgements of "true" or "false". Freud (1925a) says: "The function of judgement is concerned in the main with two sorts of decisions. It affirms or disaffirms the possession by a thing of a particular attribute; and it asserts or disputes that a presentation has an existence in reality. The attribute to be decided about may originally have been good or bad, useful or harmful. Expressed in the language of the oldest - the oral - instinctual impulses. the judgement is: "I should like to eat this" or "I should like to spit it out"; and, put more generally: "I should like to take this into myself and to keep that out". That is to say: "It shall be inside me" or "it shall be outside me". As I have shown elsewhere, the original pleasure-ego wants to introject into itself everything that is good and to eject from itself everything that is bad. What is bad, what is alien to the ego and what is external are, to begin with, identical. The other sort of decision made by the function of judgement - as to the real existence of something of which there is a presentation (reality testing) - is a concern of the definitive reality-ego, which develops out of the initial pleasure-ego. It is now no longer a question of wether what has been perceived (a thing) shall be taken into the ego or not, but of wether something which is in the ego as a presentation can be re-discovered in perception (reality) as well. It is, we see, once more a question of external and internal ". Freud words can also be applied when the reality (all the mathematical hypotheses) is not the concrete everyday reality, but an abstract formal reality that still functions according to the rules of the secondary process, i.e. of logic, and is "perceptible" only through them. However, this fictitious reality so assembled proves to be more "external" than natural reality. When studying mathematics, students are continuously faced with the problem of having to formulate judgements of the true-false type. It seems that the majority of students never reach the point of setting themselves this problem and do not spontaneously adopt any cheking procedure. Marialidia (see after) normally carried out the many arithmetic expressions that she must have come across during her lower middle school studies in the way that we have described, and she had never consciously noticed that her work was radically different from the work of her fellow-students. If students who are working in the way we have described later on, are asked to think up a proof for finding out whether the method they are using is "true" or "false", it is surprising how mentally confused they become when faced with this simple question; they do not seem able to think of an easy proof that would help them make the decision and thus avoid certain gross mistakes. For example, it would be easy to prove that the equation (a + b)3= a3+ 3ab + b3 is wrong by substituting either "a" or "b" with 1. The student's state of confusion, as indeed that of many adults long past their school days ("For Heaven's sake - the very mention of mathematics sends my brain into a spin!") brings to mind a sort of trauma due to the emotive impact with something that we know absolutely nothing about, i.e. the "meaning" of the formula. The student feels that he has to confront something "external" - the mathematical reality - that has its own specific significative organisation. But for him, the "meaning" has not been symbolically or formally contained in the formulae; it has been completely (and defensively) replaced by the formula itself.
C) ERRORS IN WORKING OUT MATHEMATICAL PROBLEMS "... the different branches of arithmetic: (Lewis Carroll, "The Mock Turtle" in Alice in Wonderland) We have often noticed that some students never manage to learn mathematics even though they would apparently like to. Teachers say about these students "He can't or won't reason", or more specifically "He's not clever enough", and they attribute the inability to learn to a lack or superficiality when studying and working, or sometimes to limited intellectual capacity. The mistakes that these students make during interrogation, tests in class or homework, suggest certain hypotheses about a mistaken and rather specific, but widespread, way of reasoning put into particular evidence by the specific structure of mathematics. Our first example differs from the others because the student concerned suffers from a serious mental handicap.
CARLO Below are some observations based on the teaching of mathematics to a young university student, Carlo, aged 23 years. The boy was an excellent high school student in the sciences, intelligent, and interested in studying. He is schizophrenic and has been in psychoanalytic therapy for about four years. The illness became evident after he had taken his high school diploma. At the suggestion of the therapist, he tried to take up his studies at university - in fact, never initiated - with the aim of sitting the mathematics exam. During the lessons certain observation were made that we shall now describe. When having to solve (a + b) (a - b), Carlo often replies that the result is 0, as though he had to add two opposite terms: -b +b = 0. One could hypothesise that his attention has focused on the fact that there is a +b and a -b rather than on the structure of the operation, i.e. a multiplication. Perhaps he has noticed only that detail - the presence of +b and -b - whiteout looking at the rest. This situation is similar to that of a small child who sees the orange-coloured skin of an orange and says that it is the sun, because he thinks that the similarity between the sun at sunset and the fruit is based only on the specification of the colour (see the case of Anna). However, Carlo manages to correct himself if he is told that it is a multiplication between polynomials; the word has to be said because merely seeing the operation is not enough for him to be able to recognise it. Sometimes, special signs have to be made such as drawing arrows in order to show the sequence of the operations: (a + b) . (a - b) = aa - ab + ab - bb (arrows) Occasionally, it is only necessary to indicate the position of the arrows with a pen for the boy to then be able to solve the problem. If two similar expressions are presented one after the other, Carlo does not necessarily solve in the same way. After a very short lapse of time, and having turned over the page, it seems as though he has completely forgotten the previous method and therefore has to start all over again. He often says: "How tiring it is!" as if he were exhausted; or else he says he has understood while, in fact, he has only read, as if he were confusing an audio-perceptive operation such as reading out loud, with comprehension and the capacity to resolve. When reading or listening to algebraic expressions, he makes certain attempts to dismantle the words, so that their meaning is destroyed. When told to write 53 (pronounced in Italian: "cinque alla terza") he writes 5a3 (in Italian "cinque a alla terza"). It is then repeated to him, clearly enunciating the difference between letters and syllables and pointing out the difference between the various sounds. But he does not seem to take this in. The two expressions are then written down and the boy is asked to read them out aloud. This may not mean anything to Carlo, so it is pointed out to him that in one of the expressions there is an "a" too many while it is not there in the other. Then he is asked to read out loud again, and sometimes he does manage to understand the difference. He often reads the two expressions in the same way so that whoever is listening does not notice any difference. In this case, Carlo has split the word "alla" (to the) into "a" (algebraic symbol) - "alla" (to the) , thus creating confusion in the meaning. One sensorial experience only - seeing the formula, or only hearing it - is not sufficient to restore the meaning, but other "signs" must be used because the integration of the five senses has been dismantled. Because he is a schizophrenic, this learning disorder is considered to be part of his far more serious mental disorder. But certain mechanisms observed in this case that could be called "autistic" according to the concept of "dismantling of the senses" described by Meltzer in "Explorations on Autism", are also present in adolescents who in all other aspects are normal. In the following example taken from the class-work of a 15-year-old student, it seems difficult to diagnose the cause of the error, and it would probably be attributed to lack of study or practice. Problem: Exercise on carrying out basic formulas of polynomial powers: (x2 - 2x + 1)2 - (x + 1) . (x - l)3 + 2 . (x - l)3 = Studentās answer: x4 - 4x2 + 1 - (x + 1) (x3 - 3x2 + 3x) + 2 (x3 - 1) = ... As can be seen, the boy solves the same equation ( x - 1 )3 first in one way: (x - 1)3 = x3 - 3x2 + 3x, so he has realised that it is a basic formula of polynomial power (even though the formula he has used is wrong), and then in another way: (x - 1)3 = x3 - 1. There is nothing to show that the boy knows that he is dealing with a basic formula of polynomial power. We wonder wether the student has realised this. Has he perhaps forgotten the rules for solving them? It is difficult to answer these questions. It seems as though the student has had a "blind spot" and has not noticed that the parts underlined are the same. It seems that his "mental faculties" have deteriorated while carrying out the exercise, and by the end he does not even recognise the basic formula of polynomial power although he appeared to have noticed it before. When students frequently make this sort of error, spontaneous doubts arise concerning their capacity for using sight and hearing in an integrated manner, or even their short and long-term memory. Therefore, the remark that has been the cause of our reflections: "These students cannot reason", can be reformulated in these terms: "These students cannot make connections; they dismantle their senses or use them in an extremely reduced and non-integrated way".
MARIALIDIA This is how Marialidia, a 14-year-old student in the first year of high school, tackles a problem in arithmetic. Problem: (- 10 + 1/20) : [ [ -0.6 - (25/2 + 9/4)] - [ -6 - (4/5 - 7/5)] ] = Correct method: -199/20 : [[ - -199/20 : [(-12 - 295) /20 + 27/5]= -199/20 : [(-307/20 + 27/5] = -199/20 : [(-307 + 108)/20]= - (-1) .(-1) = 1 Marialidia's method: (- -6/5 : [[- -6/5 : [[-1 / 63 1 / 1 : 1 = 1 ( For type reasons, in the simplification of factors, we are obliged to substitute the oblique line (/) normally used, with the horizontal line( -). This method of solving the problem is visually similar to the correct method, but it does not follow any of the rules of mathematical calculation. If the exercise were a work of art, it could be compared with Man Ray's Poem. By association, it brings to mind the "delusional reconstruction" by psychotic patients of events that are incomprehensible because they are too painful. Marialidia had done the exercises in neat, tidy handwriting, all in the same way without realising that her work was different from that of her companions and of the teacher. The answers were all completely wrong; the errors were not those typically made by students in these cases, but they were totally characteristic.
It seemed as though Marialidia did not know the meaning of the mathematical signs and symbols. She could not understand the questions she was asked in order to establish which criteria she had used to carry out the work; she seemed to have no idea of the existence of rules and operations, and said she very often got the same results as the book. The girl had been through primary and middle school, considering first arithmetic and then algebra as a mass of useless and incomprehensible rules. The only characteristic that she had taken in was the perceptive aspect of simplifying arithmetical expressions which she could reproduce like a drawing.
D) ERRORS IN THE STRATEGIES USED TO TACKLE A NEW PROBLEM Let us now look at another example. Basic formulas of polynomial powers (square and cube of binomials, square of a trinomial) are explained to the students who then carry out several exercises. On the day of the test in class, as well as exercises regarding the application of basic formulas of polynomial powers already studied, the following calculation is proposed: (a + b + c)3 (cube of a trinomial) The students immediately say that this basic formula has not been explained, but the teacher replies that with the knowledge that they already have, and by reasoning a little, they can solve the problem. Those students who think they can see a possible solution try to find it by using three methods: 1) (a + b + c)3 = (a + b + c)2 . (a + b + c) = .... result of calculations 2) (a + b + c)3 = (a + b + c) (a + b + c) (a + b + c) = ... result of calculations. 3) (a + b + c)3 = a3 + b3 + c3 + 3ab + 3ac + 3bc The first two methods can be called correct; the first is even an "elegant" solution inasmuch it allows for fewer calculations. In both cases the students realise that the formulas already known are of no use for solving the problem, but the solution can be found through the same method used to solve, for example, either: (a + b)3 = (a + b)2 (a + b) or (a + b + c)2 = (a + b + c) (a + b + c). In other words, they think that (a + b + c)3 behaves like any other binomial power that they already know, and that by carrying out certain calculations, they will be able to find a formula. The students who choose the third method, however, did not realise that the formulas they already know are of no use because, to them, a formula does not represent the abbreviation of a long, repetitive and tiresome procedure of which it constitutes the final result - useful because it is "economic" - but the formula and the basic power are the same thing. They therefore thought that in the expression (a + b + c)3 they could take the formula they knew for the square of a trinomial: (a + b + c)2 = a2 + b2 + c2 + 2ab + 2ac + 2bc and simply substitute the value of 2 with the value of 3. When asked to explain why they have done it in this way, they can only say: "I thought you could do it like this ... putting a 3 instead of a 2." Or more simply: "I thought you had to do it like this!" In their minds, mathematical procedures have no intrinsic significance, but follow law of "sequentiality" and "continuity", i.e. perceptive rules. If they are asked how to decide whether the expression they have written is right or wrong, they seem to have no idea of simple procedures that would enable them to find this out rapidly. This incapacity to supply "procedures" to find out whether an algebraic equation is right or wrong depends on the fact that the student considers the mathematical formula to be a concrete indivisible object - a concept that brings to mind H. Segal's work on the symbolic equation of psychotic thought. Sometimes, after the teacher's explanation, the students will say that they have understood. We soon realise that this only means: "For some mysterious reason, I understand that I shouldn't have done it like this", but it does not correspond to true comprehension. We could say that the student accepts the explanation from the "moral" rather than the "cognitive" aspect; he seems to consider mathematics as an elaborate system of rules, each one separate from the other (like a decalogue of good behaviour), and not as a methodology of investigation or a useful and coherent invention - for example, a language with meaning. The judgements of "right" or "wrong" based on the context are replaced by moralistic judgements or by unpleasant feelings of guilt and inadequacy. This emotional behaviour that impedes the use of explanations and corrections reminds us of certain Bionian conceptual formulations. Students who make the errors described above consider formulas as "things in themselves", separate from the meanings from which they derive. They remain in the mind as ß elements" in the disguise of undigested corpuscles - if we are to follow the alimentary metaphor so often used by teachers when students complain that they have studied hard with few results: "You have studied a lot, but you still have to digest what you have learned!" During the teachers' correction of the mistakes, these formulae = ß elements seem to take on yet another persecutory quality and become a "bizarre object" for the student: something that was difficult to understand and obscure in its deep motivations and therefore worrying in itself, is now the cause of unpleasant feelings of guilt or shame. Often, these students try to avoid explanations and clarifications about the errors they have made, for they see them as having no other purpose but to increase their suffering.
E) WAYS FOR ORGANIZING ACQUIRED KNOWLEDGE IN THE MIND We now give some examples of the ways in which students organise the knowledge they have acquired.
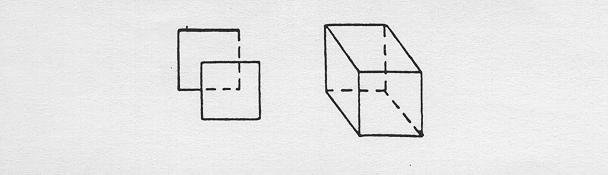
ANNA The following episode is taken from the material that emerged during the treatment of Anna, a child of just over eleven, who came into therapy because she was suffering from serious learning disorders. Although she can read and write fairly well, Anna is not able to understand the meaning of even the simplest sentences and therefore she learns nothing in any of the school subjects, especially mathematics. The episode takes place during a session about a month after the beginning of therapy. Two or three sessions before the one described, the child had decided to draw some "geometric shapes" including a cube, without a model to copy from. It can be seen that she knew the right way to draw the cube, even though she did not respect the length of the sides.
In today's session Anna finds in her box of play material four coloured wooden cubes of 3 cms. square. Two of the cubes are blue, one is red and one is yellow. She decides to draw them. She takes a page from her drawing book and places the blue cube in front of her to copy it. Looking at the cube in front of her, she makes a drawing like this:
She herself is puzzled about the result and tries to correct it with various erasures and re-drawings, but without success. After a moment of silence, she asks her therapist: "Are they cubes or dice?" ... "What's the difference?", she is asked. Anna replies that dice have spots showing the numbers. She is then told that all dice are cubes. Anna then says firmly: "Then they are cubes!" Having said the "magic word" she can now draw the cube as she has already been shown at school, using her ruler apparently to take measurements but in fact ignoring them, with the result that no surface of the cube is a square. She puts aside the blue cube and places the red one in front of her, and looking at it carefully she goes through the same procedure. She does the same with the yellow one and with the second blue cube, even though they are all of identical shape and size. She then puts the cubes she has already drawn one on top of the other, forming a tower. She colours the four figures following the orders of the colours of the cubes in the tower, and she goes over the outlines with a pen, without taking into account the visible and invisible sides. These mechanisms that are so obvious in Anna are unfortunately also present in many older so-called "normal" students who perform quite well in other subjects. Some examples: equations are explained and most students seem able to understand their concept and the procedure for solving them. Now they are presented with a question on physics or chemistry that can be solved through an equation. The majority of the students cannot recognize that the expressions written on the blackboard are an equation; and yet if the symbols are changed, substituting for example the unknown physical quantities (t = time or s = space) with x, or if the word equation is mentioned, everyone magically comes to life and they all manage to solve the problem correctly! We could say that the idea "equation and its solution" is mechanically deposited in some "place" in the mind and can be called upon only with the stimulus of certain "buttons". The concept therefore is not mobile and cannot be used as and when needed, but is fixed like a compact block. Its recognition is linked to certain perceptive parameters: denomination, form of the unknown quantity, position in space, etc. Another significant example of this phenomenon is when students manage to solve a first degree equation only if the unknown quantity is on the right hand side and not on the left, or vice-versa. Some students can solve the expression 2x = 10 straight away because they recognize it as an equation, while the expression 10 = 2x is not; they transpose the unknown quantity from right to left, changing some of the signs and thus increasing the possibility of errors, and finally they recognise something that they know and they can then solve it automatically. These forms of cognitive behaviour, as well as many others that can be observed, show us a departmentalised, mechanical and completely unintegrated way of learning, where every little piece of knowledge is separate from the others. Notions are massed together at random in areas of the mind like objects in drawers with no communication between them. This stage can be considered normal for learning a concept if it constitutes a short developmental step. In order to go on to the next stage, a mental procedure has to be confronted similar to that described by Bion as the "opacity of memory and of desire", that in this case means abandoning everything sensory; for example the writing of the unknown quantity (a, b, t, x or y), itās position on the page, etc., in order to grasp the constant conjunctions and the relational structures. In learning mathematics, one must be able to "abstain" from the sensual gratification provided by sensorial perception. Because they impose the abandoning of what is nown and concrete in favour of the unknown and abstract, mental operations of abstraction imply tolerance for the lack of the object and are themselves the cause of mental pain. We have the impression that many students who cannot bear this sensorial deprivation, try to reconstruct the concrete object that they have lost because they have had to abstain from it. The result is an absolute adherence to an ossified procedure. Very many students generally seem to prefer long, monotonous, even perceptively repetitive procedures that, because they are automatic, require little "attention" and little "reasoning", to short, concise procedures with few calculations requiring, however, an active capacity to grasp similarities, differences, an understanding of rules and a capacity for synthesis. For example, if they have to calculate the expression (x + 1)3 (x - 1)3 they proceed as follows: (x3 + 3x2 + 3x + 1) (x3 - 3x2 + 3x - 1) = x6+... thus having to carry out 16 multiplications that are long and tiresome and therefore open to errors but can be done passively, whereas they do not understand or see the advantage of being able to solve the problem much more rapidly by: (x + 1)3 (x - 1)3 = [(x + 1) (x - 1)]3 = (x2 - 1)3 = ..... They obviously want to reserve for some other purpose the attention and concentration saved by solving it in the first way. It therefore seems to us that the function of attention acquires considerable importance precisely because of its role as an "active faculty" towards the exterior. According to Freud (1911): "A special function was instituted which had periodically to search the external world, in order that its data might be familiar already if an urgent internal need should arise - the function of attention" (S.E., vol XII, p. 220). Meltzer devotes the first part of her work Explorations on Autism to the function of attention: "Most objects "receive attention". We tend to experience this kind of "unfolding" inside ourselves as an active process". For some students it is particularly difficult and fatiguing to use this "active process". It often seems that they use their mental capacity in the most "economical" way possible so that the mental contents, once perceived and codified, can "reproduce" themselves almost automatically without attention being necessary. This automatic functioning of the mind evolves towards increasing impoverishment The automation seems to crystallise and become paralysed, occupying a mental space that becomes more and more rigid. The sensorial faculties such as sight and hearing, and also the mental faculties of attention for perceiving or concentration, seem to diminish or even sometimes to be completely disappear. To an external observer, these students seem to use their minds so that they function as mechanically as possible, effecting a kind of "institutionalisation" of thought. To illustrate what happens to their minds, we may use the same words that Bion (1976) uses to describe an institution: " ... An institution behaves according to certain laws and regulations - it must establish them - and all the organisational laws become rigid and definite like the laws of physics. An organisation becomes as hard and lifeless as this table. I do not know who is able to tell at what point something that is animate becomes inanimate ... " (our translation). We can say that these students have a container-content relationship with parts of their own minds that can be defined as "very rigid" because of their tendency to "institutionalize" knowledge in this deteriorative sense. Students of this type -who are much more numerous than is commonly believed - when asked explicitly, say that they have understood what has been explained; they seldom ask questions and, paradoxically, seem to be opposed to any further explanations. They find it very difficult to connect concepts, to make comparisons, to recognise structures that are similar, and to face up to complex exercises actively and reflectively. Their difficulties increase as the science becomes more formal and greater participation and autonomous effort are required. These students, who are very passive in the sensorial and mental sense, are often very diligent; it seems as though they expect to learn by using very primitive procedures, as if they had a sort of osmosis (or form of absorption) with the scholastic situation as a whole ö an almost "parasitic" way of learning. This situation is activated especially by the group condition that prevails during the teacher's explanations, and it can be interpreted as a massive dependence coupled with magical omnipotence. Its traumatic breaking point occurs during the individual tests that the student confronts and experiences with great anxiety. Often during such situations (interrogations, exercises in class, impromptu questioning), these phenomena that we have been talking about may be attributed to a lack of sufficient attention or of logical reasoning on the part of the student; and they give the impression of dealing with a person who is not able to use his or her brain and functions properly. Is it possible that these thought mechanisms are specific to the field of mathematics and are noticeable only in these circumstances? We have no answer to this question. What is certain, however, is that the discipline of mathematics at all its various levels seems to emphasise the limit in each individual's possibilities for logical formal thought. F) DISCUSSION
Questions and comments arising from a discussion of errors can lead to hypothesising a disorder in the symbolic area of those who make the errors, that could be compared with a psychotic disorder: when faced with mathematical exercises requiring a highly symbolic performance, the subject experiences a mental pain that he/she is not able to handle. A specific defence mechanism is put into motion that avoids the pain but has a consequence: a "new reality" is constructed that is only similar to reality at the perceptive level, as in the case of Marialidia, but that ignores the "true" reality - i.e. the meaning of the formulas and of the mathematical language in general.
This could lead to the conclusion that, when faced with abstract levels, mental pain is a normal phenomenon, that the intensity of the pain varies and that there is in any case an attempt at defence against the pain, but that the modalities of this attempt can take different forms. In extreme cases the response will be bizarre, i.e. "delusion", with no perception of the strangeness. In other cases, the mistaken response tends to regress to imitative levels accompanied by a diminished capacity for judgement.
The elaboration that follows is in the form of a hypothetical genetic reconstruction. The situation of the loss of the mother, if it coincides with a feeling of necessity, is experienced by the very young child as a traumatic situation. If this necessity is not present, then there is a situation of danger. The first determining element of anxiety is therefore the loss of the perception of the object, that becomes equivalent to the loss of the object. At this stage of the genetic hypothesis, anxiety and loss exist together because the subject does not discriminate between temporary absence and definitive loss. Only after an "object" has been established and is fully characterised, and after the loss of the love of the object has acquired meaning and there are ties of desire with the object, can we refer to the reaction of pain as the actual specific reaction to the loss of the object. The reaction of anxiety, then, would correspond to the reaction of danger brought about by the loss of the object, or more directly, to the danger of the loss of the object. However, Freud cannot overlook the fact that in his thinking he has interpreted pain in various ways, and that it is not easy to establish a link between pain provoked by the invasion of a stimulus that breaks through the protective shield against stimuli, pain resulting from internal stimuli, and pain caused by the loss of an object. He appeals to the "common usage of speech [that] should have created the notion of internal, mental pain and have treated the feeling of loss of object as equivalent to physical pain" (p. 171). The continuity and the transition from physical pain to mental pain is justified in Freud's theory by making use of the concept of narcissism: this transition corresponds to a change from narcissistic investment to object investment.
There is another important emotive reaction linked to the loss of an object - that of mourning. This comes about under the influence of reality testing. Freud says that the subject himself must separate from the object inasmuch as it no longer exists. And the effort will be hard and prolonged because, through the withdrawal of huge investments, he must undo all the bonds that united him to the object. The extremely painful condition of this process is explained by the previous hypotheses. Melanie Klein (1931) who made a profound study of symbolisation disorders ("the basis of all the talents") by observing small children at play, attributed difficulties in playing and in learning to persecutory anxieties regarding sexual fantasies related to the relationship between the parents, to the combined object, and to the primal scene. For example, a learning disorder such as the inability to carry out ordinary arithmetic operations was interpreted as a defence relative to the fantasies on the sexual act between the parents. Hanna Segal (1957) considered that the non-differentiation between the symbolised thing and the symbol was caused by a disorder in the ego-object relationship; as a consequence of the conflict, the symbol becomes reduced to symbolic-equation and used to deny the loss of the object rather than to overcome it. Among the post-Kleinian psychoanalysts, W.R. Bion (1962a) in his theory on the genesis and development of thought, connected disorders in learning and in the capacity to think in general with difficulties occurring in the mother-child relationship. The problem that Bion continually poses in all his works is that of "how to deal with thoughts", or rather the proto-thoughts (sensorial and emotive impressions) that originate in the infant when he is confronted with his own internal and external perceptions - whether positive or negative. The task of resolving the question (capacity for thinking) is attributed to a special function of the mind: the "alfa" function. This function (potentially in-born and already active in intra-uterine life) acts on the sensorial and emotive impressions that "get even with" the infant in his contact with the external world, and transforms them into susceptible alfa elements of reciprocal mental connections, logical links, transformative structurisations, in such a way that these can be used as "furnishings" for dreams, daytime thoughts, memories, etc. In other words, the correct functioning of the alfa function is linked to the normal, conscious and shared relationship with internal and external reality.
But the alfa function of the newly born baby that at first can only handle pleasant impressions, must be able to develop so that it can confront negative experiences. This fundamental process of development and growth in complexity is accomplished through the contribution of the primary external object - the mother - who, with her mature alfa function and her capacity for rverie, enables the baby to confront those negative experiences due to the loss of the primary object for which he is initially unprepared. According to Bion, the baby achieves a particular type of splitting by making use of the material commodity represented by the breast without recognising its animated source. The breast, therefore, provides a service - an operation of de-animation that detaches the breast above all from its capacity to feel love and interest for the baby - the only reason why the baby is fed, looked after, etc.
Following the lines of research mentioned above, errors are placed within the defences organised in order to confront the pain caused by the loss of the primary object, and that becomes "re-actualised" when faced with a task whose character is essentially abstract and formal. For example, the defensive mechanism of "de-animation" suggested by Bion can be seen as an unconscious detachment (splitting) between the meaning and the formula representing it. The epistemophilic tension, the curiosity for the object of study, thus becomes suppressed and substituted by a mechanical manipulation of the forms considered as things in themselves and used as such, split off from their significance. Many of the errors that we came across can be considered as the outcome of this particular type of splitting and of a defective functioning of the alfa function. In the students' reasoning, concrete, perceptive elements prevail at the expense of the "significance" that is abstract and not sensorial, etc. The undigested elements pile up without form, devoid of connections (Anna, cube). Sometimes, delusional reconstructions appear (Marialidia), or the only way to proceed seems to be that of learning by heart. Often, there is an increase of physical exuberance, of restlessness used as a primitive muscular defence against mental stimuli experienced as concrete objects. Corrections and attempts to establish links become associated with unpleasant sensations of a persecutory type experienced by the student who then has the impression that he has "done something wrong" in a generic sense; sensations that are an obstacle to improvement. In Bion's words, we could say that the capacity for learning is hindered because the student seems to be dealing no longer with "mathematical objects, that is, sensorial and emotive impressions on which to exercise the factors of the alfa function", but with "bizarre objects" or elements of reality that are loaded with unpleasant persecutory connotations (fragments of the super-ego). The inability to understand can thus be described as an "attack on the link" that one part of the mind - the part that misunderstands launches against another part. The imitative, concrete and massively repetitive aspects of the incorrect procedures used, often have the "hallucinatory" function of reproducing the lost object without taking account of its loss. Mental mechanisms of various kinds can establish themselves on this so-called "basic defect" relative to the incomplete elaboration of the loss of the object. Thus, errors that appear to be mainly due to defective attention or concentration, or lack of recognition of parts perceivable as equal, seem to recall Meltzer's concept of the "dismantling of the senses". This is demonstrated very clearly - but not exclusively - in the case of Carlo. We must therefore hypothesise that the dismantling of the senses is not peculiar only to the autistic or purely schizophrenic state, but that it is a mechanism that may be activated (even in the absence of a specific pathology) in certain circumstances in which a high degree of abstraction is required. If the large number of mathematical rules (signs, parentheses, operations, etc.) that are necessary to carry out an algebraic expression are not organised in a "narrative form" through the meaning and its reciprocal links, it may possibly act on the mind like a huge mass of undifferentiated, indistinguishable and meaningless stimuli. The student then wanders from one operational hypothesis to another without being able to latch onto any of them, because on a perceptive and non-significative basis they are all equivalent or all different, as in the case of students who can solve equations only if the unknown quantity is on the left side of the equation.
At this stage, it seems opportune to explain how we have used the following expressions: H. Segal's "symbolic equation"; the term "psychotic thought" or "delusional" mechanism, etc. In the case of a small child or in other cultural contexts, these methods of reasoning may be completely normal. A child, for example, may call a large round orange the "sun", identifying it with the setting sun only because of its colour. For a certain period of time, the "names" and the "concrete things" that they denominate, coincide for the small child. The word "daddy", for instance, indicates only the child's daddy, almost like a personal attribution of the child himself (my daddy); only during a subsequent period is it perceived as a shared denomination for a series of relational and genitorial functions indicating that every child has "his" or "her" daddy. At first, the name and the physical person are the same thing because the name "daddy" does not represent a structure of particular functions and thus attributable to any person in the same condition. We know, too, that this infantile condition can be "re-produced" in certain individuals suffering from serious mental pathologies in which words and concrete objects can once again become the same thing. This seems to us to resemble the "impression" of some students that (a2 + 2ab + b2) is not the result of certain calculi and operations already present in (a + b)2 , but that (a + b)2 is equal to (a2 + 2ab + b2) as a self-accomplished identity, quite apart from the development or the structure of functional results through which, by using the same operations and functions, the student is able to find the "mathematical name" or formulae of (a + b)3 or (a + b)4 etc. As described previously, we define these methods of reasoning as "psychotic", by which we mean a particular mode of thinking or of mental mechanism that takes on certain characteristics specific not only to patients with serious mental pathologies, but also commonly present in little children or in individuals in other cultural contexts. A further example of this is when certain primitive tribes, upon the death of one of their members, cancel from their speech all the words that have anything to do with the deceased and replace them with new expressions. This method of reacting to sorrow and mourning is shared by the whole tribe and is thus consonant with individual feeling. An individual behaving in this same manner in a completely different context would encounter grave difficulty. Most people would consider it an unsatisfactory way of dealing with feelings of sorrow and mourning, and would suspect a serious mental pathology. Obviously, these same mental mechanisms when used at the collective level and in consentient contexts, do not result in any difficulties to those who use them and it is hard to understand how they could be defined as pathological. Perhaps they could better be defined as primitive or archaic ways of thinking - but this terminology, too, has certain disadvantages that we cannot go into here. In any case, by using the words "mechanism of psychotic thought" for the analogy that we have just described, we do not mean that it has any connotation whatsoever indicating a mental pathology in the normal sense of the term, for this would be misleading for our aims. The students who we are dealing with reason in a "psychotic" manner in a very particular cultural ambience - that of mathematics - and during the period of time when they confront it, and that is in high school. It is in these surroundings that they suffer all the consequences of their "mental illness", and that we are able to observe the behaviour we have described. The errors that we refer to are the outcome of ways of thinking that, if applied to reality and to normal language, would be considered symptoms of some kind of mental pathology. In reality and in the language of mathematics, they lead to errors that are not due solely to a poor knowledge of the rules, theorems or calculations, or to a lack of application, but more precisely to incorrect methods of thinking that, by analogy, we have defined as psychotic. We wish to emphasise that it is the methods of thinking that prevent these students from studying and therefore learning, and not vice versa.
What counts most for the child is The theoretical models we have mentioned above attribute the process by which the child's interest is transferred from a primary to a secondary object, both to the conflict of forces that obstruct or hinder interest for the original object as well as to actual loss of the object. In this way, the significance of the disillusion as a drive of the paranoiac or restorative type towards development and mental activity, becomes enhanced. The English psychoanalyst Marion Milner (1955), although she agrees with this line of thought, upholds the fundamental importance of the positive motivation connected with the creation of the symbol itself: greater space must be given to the modalities with which the child tries to establish a relationship with the object and with reality, besides those with which he tries to reconstruct the damaged object once a relationship with it has been established. Let us now examine the symbolic processes beginning with the first pre-logical thought and the conditions in which the primary and the secondary object are fused and felt as being one thing only; that is to say, "the moment when the original poet hidden in each one of us created our external world". Key concepts are: fantasy, inasmuch as this phenomenon can only occur in fantasy; illusion, because the person who produces the fusion believes that the secondary aspect is the primary aspect; anxiety, that is anxiety for the originary object (of retaliation or of loss) that leads to the search for the substitute; ecstasy, representing the emotive experience (the eureka!) of finding the substitute. These conditions take place in infantile play when it is used as a means to discover reality and the limit, and in the "ecstatic experiences" that the child feels in play itself.
Milner believes that the passage through ecstatic states of illusion of unity between the child's ego and the object, of contact and at the same time of annihilation of the boundary, represents a necessary and indispensable phase towards reaching and stabilising object relations. If this phase is obstructed or hindered, if the child cannot succeed in achieving detachment from primitive narcissism gradually and according to his own time rhythm, then he renounces illusion. In this case, the separation and the exigencies of necessity imposed by reality, even though apparently accepted, become a prison instead of being something with which to collaborate in order to develop greater capacities, and the symbolisation process thus becomes damaged.
The errors observed can therefore be considered not so much, or not only, as results of defensive regressive strategies due to mental pain, but rather as a blockage of the thought development process at a stage in which "normally the mind worked like this", a "prelogical" stage of thought in which the name (code) was only partially divided from the object.
When learning the mother-tongue, for instance, the mother's understanding of her child's babbling and her "giving it a meaning" form part of a fusional unity in which language begins as a mainly private communication almost exclusive to the mother and her child, as a part of play and the very early omnipotent and fusional fantasies. On the pathological side, the elective mutism of twins or of children who suffer a narcissistic trauma, bears witness to the dramatic exigencies of the symbiotic couple and throws light onto a stage of development in which subject and object are partially distinct and the "thing" and the symbol (code) coincide. In order for a second natural language to be learned, it must be affectivised either in its communicative-relational aspects or in those aspects connected to the artistic-literary production of the language itself. But while the translation of a natural language into any other language is always possible and immediately proposes a known, familiar universe that can be linked to the subject's experiences, the mathematical symbol is part of an artificial language and it "translates" into an abstract relation between abstract entities. Moreover, like the scientific disciplines, mathematics is presented - and it cannot be otherwise - in a form that is never that in which the discipline itself has developed over the centuries. The resolution of a problem whose formulation has been known since ancient times, or the organic arrangement of a theory whose complete elaboration has taken hundreds of years, is presented to the students in its final form, refined of all the erroneous trials and redundancies that had in any case contributed to reaching the goal. Every organic theory in fact recapitulates, and at the same time cancels, the previous fragmentary theories: what is gained in terms of efficiency and rapidity is evident, whereas what is lost is less obvious (Lakatos, 1976). The result is that the statement of a theory in its final form, rather than attracting and fascinating through its rigorous beauty and elegance, provokes unpleasant feelings of boredom and rejection.
This reflection leads to another. Some of the famous and creative mathematicians who left traces of how they arrived at their discoveries, describe intuitions and emotions similar to artistic or "ecstatic" experiences and processes, and these have very little to do with the elegant and concise logical reasonings proposed to students. Perhaps it is because of these determinants of the human character - especially in infants and adolescents - that for many years now in Italy and abroad, programmes and pilot projects have been developed for the teaching of mathematics. The sooner these training projects are initiated, the more valid they are; they delay abstraction and formalisation as long as possible, reaching them after a long process in which the observation and manipulation of the concrete forms, the construction of models and of the mathematical language as an internal necessity, are the fundamental stages. Inasmuch as most of the training work carried out has been demonstrated in public exhibitions, it has also been possible to see how the language of mathematics can constitute a particular reading-grid for works in the field of the figurative arts. We can therefore hypothesise that the aesthetic emotion deriving from a work of art is in some way linked to the aesthetic emotion deriving from the elegance of the mathematical theory, even though the author himself may not know that it is there. Perhaps we can suggest art as a facilitating intermediary (in the sense of our investigations) towards the learning of mathematics. It would, of course, be unrealistic to attribute to improved training methods the disappearance of the difficulties we have come across so far, even though evident changes are possible inasmuch as the causes of the thought disorders described are in any case to be sought within the modalities with which the primary mother-child relationship has been established and developed - if we are to remain within the field of psychoanalytic theories.
Although starting from completely different presuppositions, many authors and schools of thought have elaborated models and theories that in some respects have resulted in observations and conceptualisations similar to those elaborated by psychoanalysis. The cognitive school shows a certain affinity with psychoanalysis as far as the conceptualisation of defence and its mechanisms is concerned. In this field, the convergence of research acquires particular significance for our topic, because an important place is occupied by defence from the noxious stimulus. If we agree to consider the subject as a complex system of elaboration of information, without on this occasion producing any other specifications, it is not difficult to realise that he/she can come up against "elaboration disorders" that can be in the form of an elaboration that is lacking or incorrect. In this case, the causes of the disorder can be identified with desires or fears that induce the subject to avoid certain elaborations or to carry them out incorrectly. Even though research in the specific sector of the treatment of the emotions does not permit hasty conclusions, the emotive connotation of the inputs undoubtedly seems to be closely linked to elaboration disorders. Cognitive avoidance can be related to those phenomena of retroactive generalisation that explain both the loss of awareness of the avoided material as well as the particular characteristics (in psychoanalysis, of primary process) acquired by the process of elaboration itself (see M.H. Erdelyi, 1985). The result of the avoidance is then perceived in terms of a more or less serious deficit of the cognitive functions. Furthermore, through notions of defence and of perceptual vigilance, this model enables us to take into consideration well-known concepts of Freudian psychoanalysis (such as withdrawal of the attention investments) that are central to the theory of the mechanisms through which defences are carried out. They are phenomena that can successfully be simulated on a calculator. As well as this, it is also possible to introduce into the model the actual knowledge about the temporary memory register that together with selective attention has such a great influence on the processing of information.
It would certainly seem that a model such as this offers to those who, like Erdelyi, seek to relate cognitive to psychoanalytic research, the possibility of uniting the dynamic phenomena in a formalised theoretic system. It is equally evident that any system of this type leaves unresolved two questions that are essential in the psychoanalytic conception of the defence mechanisms: the variety and specificity of the different mechanisms and their semantic values. In this context, we wish to mention the work of Jean Piaget who from the time of his degree thesis in zoology (1917) until his death (1980) worked ceaselessly on the construction of a coherent design of genetic epistemology. Although controversial and perhaps in some aspects outdated, his contribution to understanding cognitive development and, in our particular case, hypothetical-deductive and formal thought development from childhood to adolescence, is of great importance. We especially refer to the book written in collaboration with the mathematician and logician E.W. Beth, Epistemologie mathématique et psychologie. Essai sur les relations entre la logique formelle et la pensée réelle (1961). As far as our topic is concerned, the specificity of this book lies in the conclusions that the authors reach, first through the development of their respective areas of competence and then in the confluence of their views according to which the problems posed by formalisation can in some way correspond with current mental mechanisms. Piaget emphasises the possible conclusion that these mechanisms co-related to formalisation come into action only in an élite of subjects (mathematicians and logicians), but also that the logico-mathematical structures are the patrimony of every subject. The actualisation of these structures in their utmost manifestations towards formalisation, would then represent a high - and considerably rare - peak in the realisation of human potentialities. Piaget's reasearch, especially in the book he wrote with Beth, considers the logico-mathematical structures leading to formalisation as "the point of arrival of a long genetic process." The mathematician and the logician, therefore, "will discover the structures that were already in action in pre-scientific thought and will then construct the corresponding theory"; this is also valid for the utmost formalisations. Also relevant is S. Ferenczi's lively, intuitive and disordered - though fertile - essay Mathematics (1920 ca.).
The views of W. Bion (1963) should also be noted in this regard. He puts the item "Algebraic calculus" right at the bottom of the grid along the axis of the genetic development of the thought apparatus. We should remember that the epistemology of the two authors is completely different: neoplatonic and neokantian in Bion, genetic in Piaget. BIBLIOGRAFY
Beth E.W. e Piaget J.
Bernardini Carlo
Bion W.R.
Bourbaki Nicholas
DSM III R.;
Erdelyi M.H.
Ferenczi Sandor
Fine R. e Fine B.
Freud Sigmund
Johnson-Laird P
Klein Melanie
Lakatos Imre
Lolli Gabriele
Meltzer Donald
Milner Marion
Paulos J.A.
Rosen V.H.,
|